TO PROFESSOR FARADAY
8 Palace Gardens Terrace, W.,
21st May 186 l.
DEAR SIR—If a sphere were set in rotation about any
diameter, it would
continue to revolve about that diameter for ever. If the
body
is of unequal dimensions it will continue to revolve about the same
axis,
provided that axis be a principal axis of the body (of
which
there are always three). If the original axis of rotation is not a
principal
axis, then the axis of rotation will change its position both in
the
body and in space.
In the body the extremity of the axis of rotation will
describe an ellipse
(or circle) about the greatest or least axis. In space it
describes
a complicated curve. The ellipse is described in
 revolutions
of the body, where 1/a^2, 1/b^2, 1/c^2 are the moments of inertia about
the principal axis.
revolutions
of the body, where 1/a^2, 1/b^2, 1/c^2 are the moments of inertia about
the principal axis.
If the body be very nearly spherical like the earth,
this mo-tion is
very slow. If P be the end of the principal axis of the
earth,
and N that of the actual axis of rotation, the rotation be-ing from W
to
E, then N will travel round P from W to E, so as to
complete
its circuit in about 325·6 mean solar days.
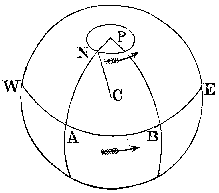
This phenomenon depends only
on the configuration
of the earth, and its rotation round an axis which is nearly
but
not exactly a principal axis, and would be the same if all other bodies
were absent from the sky. The position of the pole among the
stars
is not at all affected; but the latitude of every place on the earth is
alternately increased and diminished, the maximum latitude
occurring when N is in the same meridian with the place. Thus our
maximum
should occur sooner than that of Paris or Poulkova.
The amount of the variation can only be determined by
observation. I
have not the means at present of making refer-ence to the
statement
that Peters, astronomer at Konigsberg or Berlin, has determined the
amount
at less than 1/10 of a second, and the period about 312
days.
He has also stated the epoch of maximum at his observatory, which I do
not remember.
An explanation of this motion, illustrated by
experiments, was published
by me in the Edinburgh Transactions, vol. xxi., pt. iv., "On a
Dynamical
Top."
The motion, without reference to the earth, is
described in Poinsot’s
treatise on Rotation.
The Astronomer Royal says he may possibly be able to
test it by a long
series of observations. As far as I am concerned, you are at
liberty
to speak to any one on the subject.
I have my dynamical top in London, and can show you
the motion at any
time. I remain, yours truly,
J. C. MAXWEL


